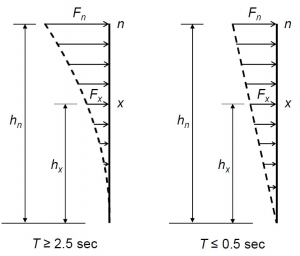
The vertical distribution of seismic forces of ASCE 7-10 Section 12.8.3 prescribes a linear distribution for structures with T ≤ 0.5 second, and a parabolic distribution for structures with T > 2.5 seconds, varying from a zero value at the base to a maximum value at the top. For intermediate periods, you can use a linear interpolation between a linear and a parabolic distribution, or simply a parabolic distribution, which is more conservative.

The linear distribution, appropriate for short-period buildings, considers fundamental mode response and assumes the fundamental mode shape to be linear. This distribution is in view of the well-known fact that the influence of modes of vibration higher than the fundamental mode is small in the earthquake response of short-period structures and that, for regular structures, the fundamental mode shape departs little from a straight line.

It has been demonstrated that although the earthquake response of long-period structures is in a large part due to the fundamental natural mode of vibration, the influence of higher modes of vibration can be significant and, in regular structures, the fundamental vibration mode lies approximately between a straight line and a parabola with the vertex at the base. The higher mode effects are adequately, indeed conservatively, accounted for when the entire design base shear is distributed parabolically along the height of a structure.

For structures having periods between 0.5 second and 2.5 seconds, a linear interpolation between a linear and a parabolic distribution of the design base shear along the height of a structure is allowed, or the entire design base shear may be distributed parabolically along the height, with a zero value at the base and the maximum value at the top. This parabolic distribution of ASCE 7-10 takes into account the higher mode effects and shifts more forces towards the top, thereby increasing overturning effects. Speaking of overturning effects, 2012 IBC Section 1808.3.1 and ASCE 7-10 Section 12.13.4 (reproduced below) are relevant to discuss at this point.

2012 IBC Section 1808.3.1 allows the seismic overturning moment, determined by the equivalent lateral force method, to be reduced by 25 percent when the foundation is designed using the strength design load combinations of Section 1605.2 or the basic allowable stress design load combinations of Section 1605.3.1, provided the structure is not an inverted pendulum or a cantilevered column type structure. This provision recognizes that there is a reduction in overturning moment caused by the higher modes of vibration, because in any mode of response other than the fundamental mode, all the masses do not move in the same direction. There is also a reduction in response due to the effects of uplift at the foundation-soil interface; it ought to be remembered that the equivalent lateral force analysis of Section 12.8 assumes a fixed base of the building. No reduction is permitted for an inverted pendulum or a cantilevered column type structure because structural stability depends entirely on resistance to overturning moment at the base and because there is no redundancy in the system.

The alternative basic ASD load combinations provide a lesser margin of safety against overturning, compared to that provided by the basic load combinations for strength design and allowable stress design. Therefore, a reduction in foundation overturning moment is not permitted with the alternative basic ASD load combinations.

The reduction in overturning moment at the soil-foundation interface is only 10 percent for foundations designed in accordance with the modal response spectrum analysis procedure. A lower reduction in the overturning moment obtained using this procedure is justified because the reduction in response due to higher mode effects is already accounted for in the analysis.